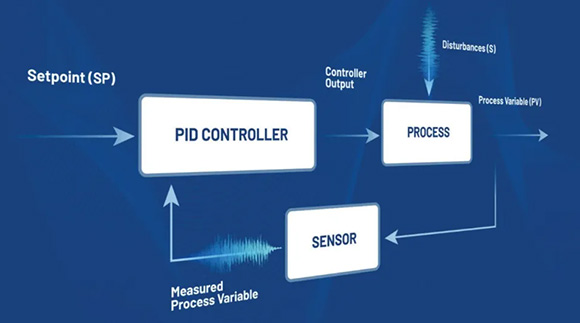
Back in collegewww.cechina.cn, I learned that the ubiquitous proportional-integral-derivative (PID) control algorithm consists of three operators or terms acting on the error signal as shown in the “Theoretical PID algorithm” graphic. The Controller calculates the integral and derivative of the error between the process variable and setpoint控制工程网版权所有, then generates a control effort by adding those two quantities to the error itself. A user chooses the tuning parameters—proportional gain (P), integral gain (I) and

derivative gain (D)—to give more or less weight to each term, thereby adjusting the controller's performance.

　　The corrective effort that a PID controller applieds to a process is the sum of the proportional action generated by the proportional term (top), the integral action generated by the integral term (middle), and the derivative action generated by the derivative term (bottom). The PCONTROL ENGINEERING China版权所有, I and D tuning parameters determind the relative contribution of each term. This arrangement is also described as the non-interactiong or independent algorithm since changing one tuning parameter does not affect the contributions of the other two terms.
　　I proceeded to write my first PID tutorial for Control Engineering assuming that commercial PID controllers work just that way. I soon learned that commercial PID controllers do compute the error signalwww.cechina.cn, its integral and its derivative, but they typically combine those three quantities using the standard form of the PID algorithm as shown in the “Standard PID algorithm” graphic. It accomplishes the same end result as the theoretical algorithm, but it uses a slightly rearranged PID equation with a different set of tuning parameters – controller gain (Kp), integral time (Ti) and derivative time (Td).
　　Potential for confusion
　　Fortunately控制工程网版权所有, it's easy enough to compare the two algorithms and relate PCONTROL ENGINEERING China版权所有, I and D to K PCONTROL ENGINEERING China版权所有, T i and T d as follows:
　　P = Kp
　　I = Kp / Ti
　　D = Kp · Td
　　The proportional gain P is the same as the controller gain K PCONTROL ENGINEERING China版权所有, s